Mathematician – By's Musings

An 1810 pencil and paper sketch of Archimedes by an unknown artist. The original is located in the Museum of Fine Arts in Rio de Janeiro. As a photographic image of a two-dimensional work of art in the public domain, it is also in the public domain. Image courtesy of Wikimedia Commons and the Museum of Fine Arts, Rio de Janeiro.

Some might claim that the two phrases that I used to describe Archimedes in the title of this post say the same thing. Even though mathematicians have the reputation of being strange, I am honored, but humbled to claim the mantle of a mathematician. The roles and duties of a mathematician are to identify and solve problems, to quantify and count those things that can be enumerated, to unfold and qualify those things which can’t be enumerated, and to discover and disseminate the beauty of patterns within this world.

I know my work as a mathematician is overshadowed by many great mathematicians like Archimedes. It is still my calling. Image courtesy of Presenter Media.

It is a big job. However, I was called to this vocation and I love it. I know that my mathematical accomplishments will hold a candle to those of Archimedes. Nevertheless, I count it a privilege to work in the shadow of Archimedes, Pythagoras, and so many others.

According to the many legends about Archimedes, the opening sketch shows him in what would have been a typical pose for him: deep in thought pondering a mathematical, geometric, or engineering problem, tinkering with some toy, piece of equipment, or prototype. Archimedes was the archetypical professor, preoccupied in his thoughts to the exclusion of everything else.

Map of Sicily c 431 B.C., showing the city of Syracuse of its Eastern coast. Abu America, copyright holder has licensed its use under the Creative Commons Attribution-Share Alike 3.0 Unported license. Image courtesy of Abu America and Wikimedia Commons.

It is believed that Archimedes was born sometime in the year 287 BC, in the city of Syracuse on the island of Sicily. His father, Phidias, was a well-known astronomer and mathematician, and a relative of the King of Syracuse. As a young child, Archimedes was tutored by the best teachers in Italy. In his teen years, he reportedly traveled to Egypt and studied under their greatest teachers, mathematicians, scientists, and engineers. In his early twenties, he returned to Syracuse where he lived the remainder of his life, solving problems and corresponding with his Egyptian colleagues.

Engraving of Archimedes moving the world with his lever from Mechanic’s Magazine (cover of bound Volume II, Knight & Lacey, London, 1824). It is in the public domain because it was published prior to 1924. Courtesy of Wikimedia Commons and the Annenberg Rare Book & Manuscript Library, University of Pennsylvania, Philadelphia

I first became acquainted with Archimedes as a fourth-grader, when our teacher introduced the topic of levers during a science lesson on simple tools. The teacher began his demonstration by using an exaggerated statement he attributed to Archimedes: “Give me a place to stand and a lever long enough, I can move the world.”

In the mid-1950s, at least five years prior to human space travel, we all knew that this was a physical impossibility. But it actually made some sense, as we constructed our own levers to move objects.

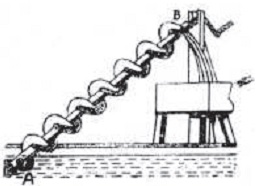
Archimedes’ screw has been used for centuries as a means of transferring water from a low-lying body to a higher level. This example is a sketch of a working screw from the University of Mysore, Karnataka, India, which has licensed the image under the Creative Commons Attribution-Share Alike 3.0 Unported License. Image courtesy of Wikimedia Commons and University of Mysore

By the time our teacher introduced the Archimedes screw in a subsequent lesson as an example of another simple tool, I was hooked. I was an Archimedes fan.

This guy rocked. One year earlier, I had “helped” my father build an automatic stoker for the coal furnace in our house using an auger that he bought from a farmer that no longer needed it to carry feed to the milking herd that he had sold. That auger was an exact replica of Archimedes’ screw. My father used it to move coal horizontally rather than moving water vertically.

Diagram of Archimedes Method for Solving Problems. Chart created by blog’s author using Click Charts software.

As our teacher told us more about Archimedes, I found that he would take both seemingly simple and complex, real-world problems and use the principles of engineering, physics, and mathematics to solve them. As our teacher systematically detailed the six steps in Archimedes’ method for solving problems, they were indelibly fixed in my mind.

Although I may have demonstrated some of the engineering brilliance of Archimedes, I haven’t yet talked about any of his mathematical work or any of his eccentricities. What were some of the mathematical problems that Archimedes attacked and solved?

It was well known by the time of Archimedes that there was a constant relationship between the radius of a circle and both its circumference and area. We now know this constant as pi or π. Although the use of the Greek letter π was not adopted for general use until the 18th century AD, for the sake of brevity and clarity I will use it for the remainder of this blog. Since Archimedes was generally considered the first to document an attempt to approximate this constant as closely as possible, it is sometimes called Archimedes’ constant.

By 1900 BC, Babylonians had estimated that π was approximately 25/8. In the same time period, Egyptians began using a value of 256/81. The decimal representation of fractions can be traced back to China by the 4th century BC, before slowly spreading through Asia, to the Middle East, and then to Europe. Europeans didn’t fully adopt this approach to expressing non-integral values until they had embraced the base-ten, positional Hindu-Arabic number system that we use today. Thus, we can say that the Babylonians estimated that π was approximately 3.125, while the Egyptians estimated that π was approximately 3.1605.

This image of Solomon’s Temple shows the great basin in the left foreground. As a faithful reproduction of a two-dimensional work of art in the public domain, it is in the public domain. Image courtesy of Wikimedia Commons.

A millennium later we find the ancient Israelites using “3” as an estimate of π in a Biblical reference related to the design of Solomon’s temple.

And he made a molten sea, ten cubits from the one brim to the other: it was round all about, and his height was five cubits: and a line of thirty cubits did compass it round about. (I Kings 7:23, KJV)

The molten sea was actually a large bronze basin filled with water meant to symbolize the chaos of the world during creation when God’s spirit moved upon the waters.

And the earth was without form, and void; and darkness was upon the face of the deep. And the Spirit of God moved upon the face of the waters. (Genesis 1:2, KJV)

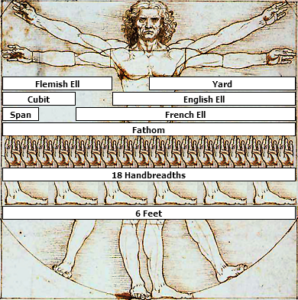
his derivation of the Vitruvian Man by Leonardo Da Vinci depicts nine historical units of measurement: the Yard, the Span, the Cubit, the Flemish Ell, the English Ell, the French Ell, the Fathom, the Hand , and the Foot. Da Vinci drew the Vitruvian man to scale, so the units depicted here are displayed with their proper historical ratios. The creator of the work and the copyright holder, Unitfreak, has released the work into the public domain. Image courtesy of Unitfreak and Wikimedia Commons.

Simple mathematics would tell us that if the diameter of the basin was 10 cubits and its circumference was 30 cubits, the ratio of circumference to diameter was “3.” This is “short” of our current value of π. However, there is a “fudge” factor built into the biblical account. A cubit was never a precise measurement. A cubit was generally considered the length of the forearm of a typical man which is the distance from the elbow to the tip of a man’s middle finger. It was generally about 18 inches or 44 centimeters. However, it could vary with each person making the measurement.

This imprecision didn’t sit well with mathematicians or builders. Although a few mathematicians worked on this problem, it took almost another millennium for Archimedes to come up with a different approach to the problem.

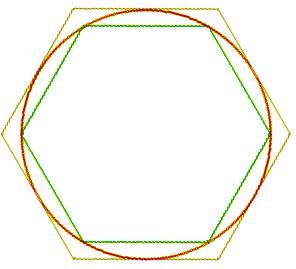
The starting point of Archimedes’ calculation of π is a circle of diameter 1, with inscribed and circumscribed hexagons. This image was uploaded to Hebrew Wikipedia by דוד שי who licensed its use under the Creative Commons Attribution-Share Alike 3.0 Unported license. Image courtesy of דוד שי and Wikimedia Commons.

Archimedes tackled the problem of calculating the circumference of a circle using an iterative approach and an over-and-under perspective. He inscribed and circumscribed hexagons inside and outside a circle with a diameter of 1. He was able to calculate the circumferences of the two hexagons, using only the following two results:

1. Pythagorean Theorem: The square of the hypotenuse of a right triangle is equal to the sum of the squares of the other two sides.

( a² + b² = c² )

2. Proposition 3 of Book IV of Euclid’s Elements: If an angle of a triangle be bisected and the straight line cutting the angle cut the base also, the segments of the base will have the same ratio as the remaining sides of the triangle; and, if the segments of the base have the same ratio as the remaining sides of the triangle, the straight line joined from the vertex to the point of section will bisect the angle of the triangle.

Using these two results Archimedes converted the geometric problem to strictly an algebraic problem, howbeit a complicated one. He then iterated the process by successively doubling the number of polygons which inscribed and circumscribed the circle with a diameter of 1. By the time he was using 96 polygons, he had narrowed down the upper and lower limits of π to 3.1408 and 3.1428. This approximation is accurate to two decimal places, which is accurate within 0.4%.

Archimedes’ sphere and circumscribing cylinder. This image was created by André Karwath. It is licensed under the Creative Commons Attribution-Share Alike 2.5 Generic license. Image courtesy of André Karwath and Wikimedia Commons.

The mathematical problem that Archimedes claimed to be proudest of solving was finding the volume and surface area of a sphere. Archimedes showed that the volume of the sphere has 2/3 the volume and surface of the circumscribing cylinder. Thus, the surface area and volume of a sphere with radius r are given by the equations:

A = 4 πr²

and

V = (4/3)πr³.

Cicero discovering the tomb of Archimedes in an 1897 painting by Benjamin West. As a faithful reproduction of a two-dimensional work of art in the public domain, it is in the public domain. Image courtesy of Bridgeman Art Library and Wikimedia Commons.

Archimedes was so proud of this mathematical accomplishment that he requested that a cylinder encasing a sphere adorn his tomb when he died. This is how the tomb of Archimedes was eventually identified.

In 75 BC, 137 years after Archimedes’ death, the Roman statesman and philosopher Cicero in his Tusculan Disputations Book V, Sections 64 – 66, wrote the following:

When I was questor in Sicily I managed to track down his grave. The Syracusians knew nothing about it, and indeed denied that any such thing existed. But there it was, completely surrounded and hidden by bushes of brambles and thorns. I remembered having heard of some simple lines of verse which had been inscribed on his tomb, referring to a sphere and cylinder modelled in stone on top of the grave. And so I took a good look round all the numerous tombs that stand beside the Agrigentine Gate. Finally I noted a little column just visible above the scrub: it was surmounted by a sphere and a cylinder.

Probably the most famous incident that illustrated Archimedes’ single-mindedness and his eccentricities involved a royal crown and a bath. Archimedes was the go-to-guy in ancient Syracuse to solve problems. Thus, when the Hiero II, King of Syracuse was faced with the suspicion that he had been cheated by a dishonest artisan, he asked for Archimedes’ help.

Heiro II commissioned the creation of a solid gold crown to adorn statues of the gods and goddesses of Syracuse. Since the crown was to be part of a worship activity, Heiro didn’t want to offend the divines. He had to be sure that it was really solid gold, but he couldn’t melt down the crown to find its makeup. So he asked Archimedes to determine a way to test the crown without disturbing or disfiguring it.

Woodcut print from the book “Historical and critical information about the life, inventions and writings of Archimedes of Syracuse” by Count Giammaria Mazzuchelli (1707-1765), published in Brescia, Italy in 1737. As a faithful reproduction of a public domain work, it is in the public domain. Image courtesy of Wikimedia Commons.

In the most famous legend associated with this storying Archimedes was thinking about how to solve this problem while he was taking a bath in one of the public baths of the day. According to the legend, as Archimedes began to submerge himself, he realized that his body mass was displacing an equal mass of water. Here was the solution. Archimedes suddenly screamed “Eureka!” and jumped up and ran home to test his solution. In his haste, he forgot his clothes, so supposedly he was running through the streets of Syracuse naked, yelling “Eureka!” which means “I found it!“

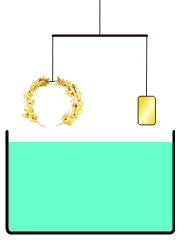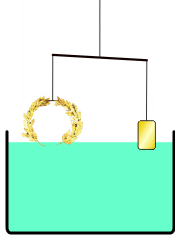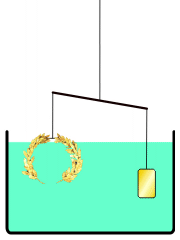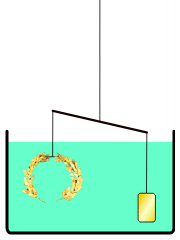
As gold mass equal in weight to the crown are submerged, if the crown rises toward the surface, it has less gold in it.

Although there are many versions of this story, I tend to believe the following one is the most plausible. This version is attributed to Vitruvius, a Roman architect of the first century BC. The Archimedes buoyancy principle may have been used to determine whether the golden crown was less dense than gold. Given that both the crown (left) and the reference weight (right) are of identical volume, the less dense reference weight object will experience a larger upward buoyant force, causing it to weigh less in the water and float closer to the surface.

This depiction of the Claw of Archimedes from a 17th-century wall painting by Giulio Parigi expresses visually uses the literal translation of the Italian word for claw as a giant iron hand. As the faithful reproduction of a two-dimension work of art in the public domain, it is in the public domain. The original is in Room 17 of The Stanzino delle Matematiche of the Uffizi Gallery in Florence. Image courtesy of Uffizi Gallery and Wikimedia Commons.

When Syracuse was attacked by Roman naval forces, King Heiro II also commissioned Archimedes to invent weapons to defend the city. According to the legends, Archimedes responded with two monstrous creations. The first was known as the Claw of Archimedes.

It was a giant crane with a long line with a hook that caught the bow of any ship that ventured too close to the seawalls defending Syracuse. By raising and lowering the crane arm, it was able to swamp and/or overturn the boat caught by the hook. It only took one or two encounters with this weapon for the Romans to learn to stay far enough away from the seawall.

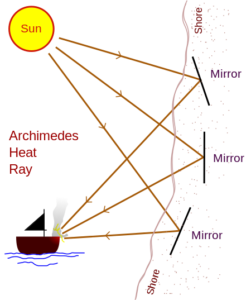
Conceptual drawing of Archimedes Death Ray using polished mirrors to concentrate enough sunrays to set Roman ships afire. The drawing is by Finnrind and is licensed under the Creative Commons Attribution-Share Alike 3.0 Unported license. Image courtesy of Finnrind and Wikimedia Commons.

With the Roman boats now staying a bowshot away from the shore, Archimedes was challenged to come up with another weapon to defeat this persistent enemy. This led to the legend of Archimedes’ Death Ray. Most every small child knows that using a magnifying glass or a parabolic mirror, one can start a fire from the sun’s rays. According to the stories, Archimedes used this principle to set the Roman ships afire.

Over the intervening centuries, many experiments have been done to try to recreate the incendiary events. In all cases, more mirrors than shown would be needed, and the usual results may have been temporary blindness and confusion among the sailors rather than fire.

Since there are Roman accounts of ships burning and sinking, something probably happened. The mirrors may have consisted of polished metal and had peep-holes drilled in the middle for use in aiming. Whether they set the Roman ship afire, or just created enough confusion so that small crafts from the defenders of Syracuse could get close enough to fling burning vats of “Greece fire,” i.e., burning oil, will never be known.

The Roman commander Marcellus knew through the tales of spies and traitors that the machines that Archimedes had invented caused the problems for his troops. He laid siege to the city and after a protracted period of time, finally wore them down due to the lack of supplies. He ordered his troops to destroy everything and everyone with one exception. Under no circumstances was Archimedes to be harmed. He was to be taken alive. Marcellus wanted this genius working for him.

A print from the biography of Archimedes written by Giammaria Mazzucchelli from 1737. This is a faithful reproduction of a two-dimensional public domain work of art contained in the Vatican Library. Thus, it is in the public domain. Image courtesy of the Vatican Library and Wikimedia Commons.

Here is where the single-mindedness of Archimedes got the eccentric in trouble again. A soldier found Archimedes working on a mathematics problem drawing circles in the sand. The soldier ordered Archimedes to come with him. Archimedes told the soldier he couldn’t because he was in the middle of solving an important problem. The soldier started scratching out the sand circles. Archimedes yelled at the soldier “Nōlī turbāre circulōs meōs!” a Latin phrase, meaning “Do not disturb my circles!”

The soldier got angrier with Archimedes and killed him. When this was reported to Marcellus, he ordered the soldier killed, and Archimedes to be given a burial befitting royalty. Hence the fancy tomb that we had noted that was discovered by Cicero. Archimedes was indeed an eccentric, as well as a mathematical and engineering genius.

In my next post, I will return to considering some of the crisis facing modern American higher education. The next crisis on the agenda is the crisis centered on and created by the Faculty Reward Systems and Faculty Priorities. I hope to post it next week.

This is a modified page scan of “The Ugly Duckling” a story from “Fairy tales and stories” by Hans Christian Anderson, translated by Hans Lien Brækstad, with illustrations by Hans Tegner. The book was originally published in 1900. and as such is in the Public Domain. Image is courtesy of Wikimedia Commons.

From the comments I received after I published the recent post Are All Mathematicians Crazy?, it is obvious that I didn’t convince many readers that some mathematicians are almost normal. I readily admit that some mathematicians are off the chart on the eccentric side of the normality continuum. These famous curve busters make it difficult for the rest of us. There are mathematicians who indeed were strange birds and didn’t always fit the normal mode. They were different and stood out from their peers. Through the years, these unconventional mavericks have gotten most of the press coverage. Once you admit that you are a mathematician, you are automatically branded as different.

In a series of posts, I will discuss some of the more stranger mathematicians among us. The earliest outlier that I want to discuss is Pythagoras of Samos (c. 570 – c. 495 BC). He has been called by many the leading philosopher and ethicist of his day. Since there is no record of Pythagoras ever putting quill to papyrus, we have none of his works in his own words. All that we know of him and his teachings are what others have recorded. If half of the legends concerning Pythagoras are true, then in today’s vernacular, he could easily be labeled “a strange duck.”

Cropped photograph of the bust of Pythagoras in the Vatican Museum. Pythagoras is portrayed as a tired old man. This photograph was uploaded to English Wikipedia by Andargor in March 2008. Andragor released the image to the public domain. Image courtesy of Andragor and Wikimedia Commons.

Although he influenced many great philosophers, ethicists, and mathematicians, he is probably best known for the formula bearing his name, the Pythagorean Theorem, which states that the square of the hypotenuse of a right triangle is equal to the sum of the squares of the other two sides. However, Pythagoras didn’t discover this formula since it was used in construction in Sumerian and Egyptian civilizations at least one millennium before he lived. It bears his name because Pythagoras is credited with the first generalized proof of this relationship, which is the proof I referenced in my previous post Are All Mathematicians Crazy?.

In terms of Pythagoras’ life, there are many contradictory stories. We believe he was born on Samos, a Greek Island in the Aegean Sea near modern day Turkey. His father most likely was a European merchant living and trading on Samos. Legend has it that Pythagoras, as a child and young man, traveled extensively throughout Asia, Asia Minor, Europe, and Africa. He reportedly sat under the tutelage of the best teachers and priests in Asia Minor, India, Egypt, and Greece.

Photograph of a page from the book “The Story of the greatest nations” by Ellis and Horn, published in 1913. The scene depicts the Pythagorean School in Croton. Since the book was published before 1924, it is in the Public Domain. Image courtesy of Wikimedia Commons.

We know that he was a gifted thinker and a great teacher. People traveled from all over the world to sit at his feet and learn from the master. He started a school known as the Semicircle since that was the shape of the Pythagorean classroom. This classroom model is still very common in college settings today. Pythagoras would take center stage with a clear view of all students, while the students could see all other students and direct answers and questions directly to them as well as the teacher. However, students have always been students. Notice the lack of attention on the face of the one student in the foreground, staring off into space. Even the great teacher Pythagoras couldn’t keep her attention.

In the illustration of the Pythagorean School, most of the students depicted are women. Pythagoras was the first Greek philosopher or teacher who advocated education for women. He was also the first prominent Greek to promote monogamy within marriage. His influence on women’s rights and the education of women was felt for centuries.

From his time in an Egyptian temple, he may have picked up his ideas on metempsychosis, the belief in reincarnation. It is reported that Pythagoras could recall all of his former lives. He entertained his students and followers for hours on end with stories of his former lives. Since he believed that he was there in one of his former lives, he supposedly enthralled his listeners with vivid accounts of the great battle and fall of Troy. In at least one incarnation, Pythagoras was supposedly a beautiful courtesan, a prostitute who lived an unhappy and unfulfilled life in the lap of luxury, courtesy of her wealthy customers. Some writers attribute his high regard for women to the time he spent as this oppressed woman with few rights.

A photograph of the Temple at Luxor in 1867 by Félix Bonfils. This image is in the public domain since Bonfils died in 1885 and the copyright subsequently expired. Image courtesy of Wikimedia Commons.

While in Egypt, it has been reported that he was admitted into the priesthood at the Temple of Karnak near the cities of Thebes (Greek name: Diospolis – city of the gods) and Luxor. If true, he would have been the only non-Egyptian to have ever been granted this great honor. Supposedly he learned much of his geometry from the Egyptian priests. They also instilled in him their lifestyle and moral codes, which included abstinence from sexual pleasure, and avoidance of clothes made from animal skins. The Theban priests were vegetarians with one quirk. They refused to eat or even touch beans. This unusual behavior was apparently well-engrained into Pythagoras.

Pythagoras studied at Luxor for ten years until Cyrus and the Persian army defeated the Egyptians in 526 BC. In the battle for Thebes, the Persians killed Egyptian Pharoah Psamtik III, son of Amasis II. The Persians were so enamored by the size and beauty of the Luxor Temple that they ordered the defeated Egyptians to rebuild Thebes and repair all damages to the Luxor Temple. The Persians were also impressed with the intelligence of Pythagoras, a Greek they found among the priests at Luxor. They took him captive back to Babylon, where he studied under the wisest sages of Persia for another ten years.

Photograph of a page from an early 16th Century French manuscript drawn by an unknown artist using a pen, brown ink and watercolor on paper. It depicts Pythagoras repulsed by fava beans. The manuscript was a gift of Andrea Woodner to the National Museum of Art, Washington, D.C. Image courtesy of the Woodner Collection, National Museum of Art, and Wikimedia Commons.

Many historians believe that after leaving Egypt, Pythagoras had a life-long battle with an irrational fear of beans. If the legends are correct, his leguminophobia may have cost him his life. Years later when his school at Croton in Italy was attacked and destroyed, Pythagoras supposedly escaped. While running away from the attackers, he stumbled upon a field of beans. He froze in his tracks and would not go any further. According to one legend, the rioters found him terror-stricken, cowering at the edge of the field he had refused to enter. They proceeded to beat and club the old man to death.

According to a second legend, the rioters knew Pythagoras was deathly afraid of beans. Thus, they never searched for him in the vicinity of the bean field because they knew that Pythagoras would have never approached it. After hiding in the weeds on the edge of the bean field for a long time, Pythagoras returned to his school. Seeing that it was destroyed and many of his students killed, he left Croton for Metapontum to escape persecution for his anti-democracy teachings. In Metapontum, he supposedly hid in the Temple of the Muses. He reframed from eating because the priests of the Temple didn’t provide the vegetarian diet he requested. They offered him meat and beans. After 40 days of a self-imposed hunger strike, he died of starvation in the temple.

There are many other stories and legends of the exploits of Pythagoras. If only a small fraction of them were true, Pythagoras was indeed different and could be considered a strange duck. The next unusual mathematician that I will consider is Archimedes. In the meantime, I will return to my series on the changing scene in American higher education.

Archimedes: A Great Mathematician, But Very Eccentric

Some Mathematicians Are Different